

We can see just by this approximation that we’ve drawn in that 𝜃 will be bigger than 28 degrees. If we did shift that vertex, so that it now actually is at the centre of the protractor, then see what that does to the value we’d read off for 𝜃. The fact that that hasn’t been done will lead to and has led to a flawed reading of the angle 𝜃. We need to align those two points, the centre of the protractor and the vertex of the angle. And in fact, that’s the first step we take when we want to measure an angle using a protractor. The centre of our protractor right here and the position of the vertex of our angle over here are separated by some distance. We can tell right away that this is at least a true statement. And that vertex is right here, where the two rays meet. This leaves us with answer option C, which tells us that the reason that this value of 28 degrees is not a correct reading for 𝜃 is that the centre of the protractor, that’s right here marked out by this cross, was not placed at the vertex of the two lines that form the angle 𝜃.
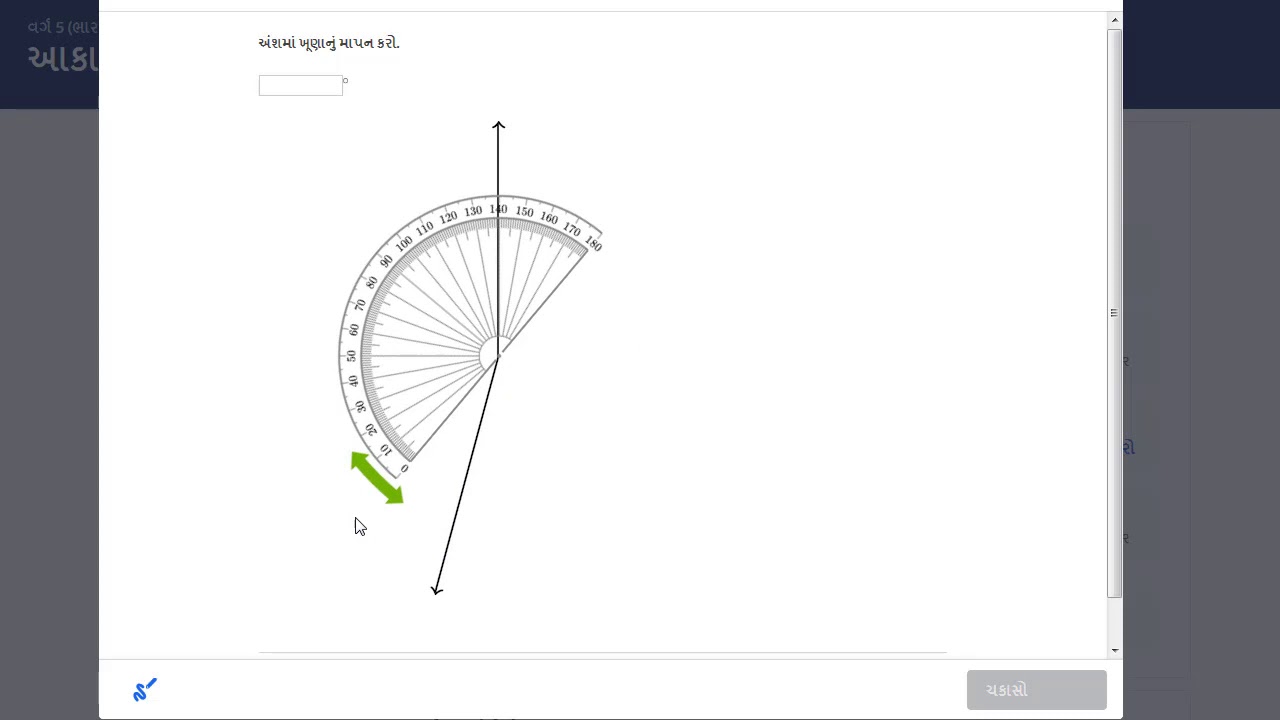
That tells us that neither A nor B is a correct answer option. Notice that neither A nor B offers this is as the resolution of the protractor. That tells us that the smallest division of this protractor is one-tenth of that or one degree. In all then, we’ve counted 10 even divisions between this change of 10 degrees on our protractor. And then our 10th hash mark is 70 degrees, one of the larger ones. If we were to move up our angular scale counting hash marks as we go, we would count one here, then two, then three, then four, then five, with the medium-sized hash mark, then six, seven, eight, then nine. And just to make our analysis a bit easier, let’s work off of the 60-degree scale, that is, the one that starts at zero on the left-hand side of the protractor. Say we start with this one, which is at 60 degrees or 120 degrees. To figure out what that resolution is, we can start with one of the larger hash marks on our protractor. That’s the smallest possible angle it can tell the difference between. We see that answer options A and B both have to do with the resolution of the protractor. From our different answer options, we want to pick which one correctly explains why that value is not right. We’re told that the reported value of this angle is 28 degrees and that that is incorrect. That angle is defined by these two rays right here, also called sides of the angle or arms. Okay, taking a look at our diagram, we see we have a protractor and an angle labelled 𝜃. C) The centre of the protractor was not placed at the vertex of the two lines that form the angle 𝜃. B) The maximum resolution of the protractor is 10 degrees, so the angle should be measured as 30 degrees. Which of the following statements explains why this answer is incorrect? A) The resolution of the protractor is 0.5 degrees, so the angle should be measured as 27.5 degrees.

Chloe uses a protractor to measure the angle 𝜃, as shown in the diagram.


 0 kommentar(er)
0 kommentar(er)
